Underground fluid interactions take place between miscible fluids (oil and miscible CO2) and immiscible fluids (water and oil). Many factors determine the transport of fluids, one of which is fluid mixing.
In most conditions, mixing in a reservoir is due to a combination of advective transport and diffusive transport. The ratio of the rate of these two transport mechanisms, known as the Peclet number, can be used to characterize flow in a reservoir and in Interface’s microfluidic systems.
Peclet number formula
The Peclet number is a dimensionless number defined as the ratio between the rate of advection to the rate of diffusion. It is given by:
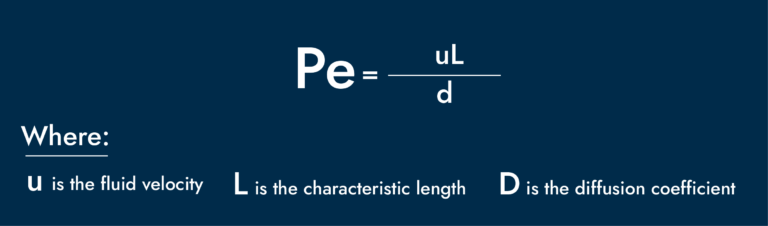
Diffusion matters
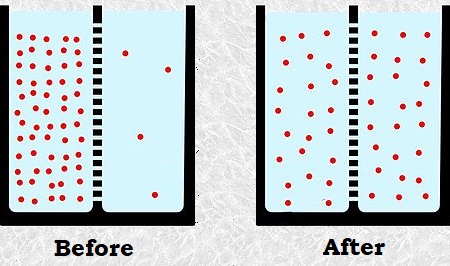
Diffusion is the net movement of matter from a region of high concentration to a region of lower concentration. In a liquid-liquid diffusion process, there is no net movement of liquid, only a gradual change in concentration due to a concentration gradient.
This figure shows the initial state of a system with a concentration gradient. Over time the red molecules will move into the area with low concentration due to diffusion.
When two miscible fluids meet each other underground, chemical species from each fluid begin to diffuse into each other. Diffusion alone is very slow; some tests Interface Fluidics ran for Total showed that propane can take four minutes to travel one mm into heavy oil (0.4 m/day).
So, when one is mixing sugar into a morning cup of coffee, it’s good to rely on more than diffusion alone.
Measuring the diffusion coefficient of reservoir fluids
Microfluidics can be used to accurately measure diffusion by creating a system with no bulk fluid flow. A simple system for analyzing diffusion was developed in Professor Dave Sinton’s Lab at The University of Toronto. Interface has adapted this system for use at high pressures and temperatures relevant to over 80% of the world’s reservoirs.
Dr. Sinton’s design allows for a fresh boundary to be created between two liquids and then to stop the flow in the system so that diffusion can be observed. This is also valuable in CO2 diffusion studies for carbon capture and storage.
In another example, the diffusion of propane into heavy oil was studied. As propane diffuses into heavy oil, the fluorescence changes. This change can be compared to a calibration curve, and the rate of change in concentration over time can be used to determine the diffusion coefficient.

Interface has extensive experience working with heavy oil, not only in diffusion studies but also through our steam-assisted gravity drainage fluid performance screening. Through this service, we assist clients in selecting the best solvents, alkaline additives, and non-condensable gases to augment their injected steam.
In solvent-based SAGD testing, diffusion usually takes place between the two miscible fluids, which Interface is able to optically observe and analyze at the pore scale.
All about advection
Advective transport is the bulk movement of fluid due to its velocity. It is often used synonymously with convective transport, which is the bulk movement of fluid due to density gradients (often created by thermal gradients).
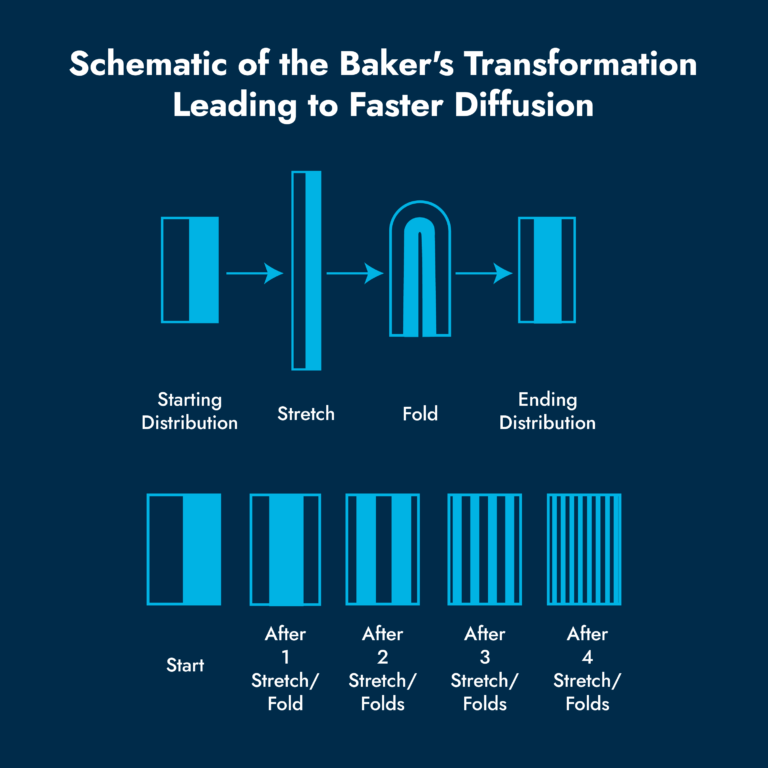
Advective mixing takes place due to the bulk movement of two fluids relative to each other. It creates more contact area between two miscible fluids and reduces the length-scale that diffusion takes place. This results in faster mixing and is more dominant the larger the scale of the fluid system.
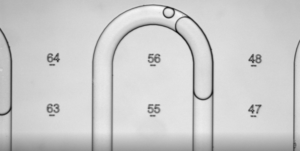
Here, we’ve illustrated the concept of the baker’s transformation, where repeated folding and stretching creates narrower, more numerous pathways for the fluid. This significantly accelerates diffusion as it allows for a greater contact area over which it can take place. Resulting in shorter length scales and faster mixing.
This image is an example of a more modern microfluidics-based fluid mixer created by Interface. Here oil (white) and hexane (black) come in as two separate phases. As they traverse the device, a similar sort of folding of the fluid takes place, which results in accelerated mixing via diffusion. By the end of the test, the two fluids form a homogeneous (grey) mixture.
In a diffusion-dominated system, the Peclet number is less than 1. In an advection-dominated system, the Peclet number is greater than 1. Most systems in microfluidics have very small length scales and very small fluid velocities, so the Peclet number is usually small.
The exception to this is when the microfluidic system is designed in such a way that advective transport is forced to be dominant, like in Interface Fluidics’ minimum miscibility pressure test.
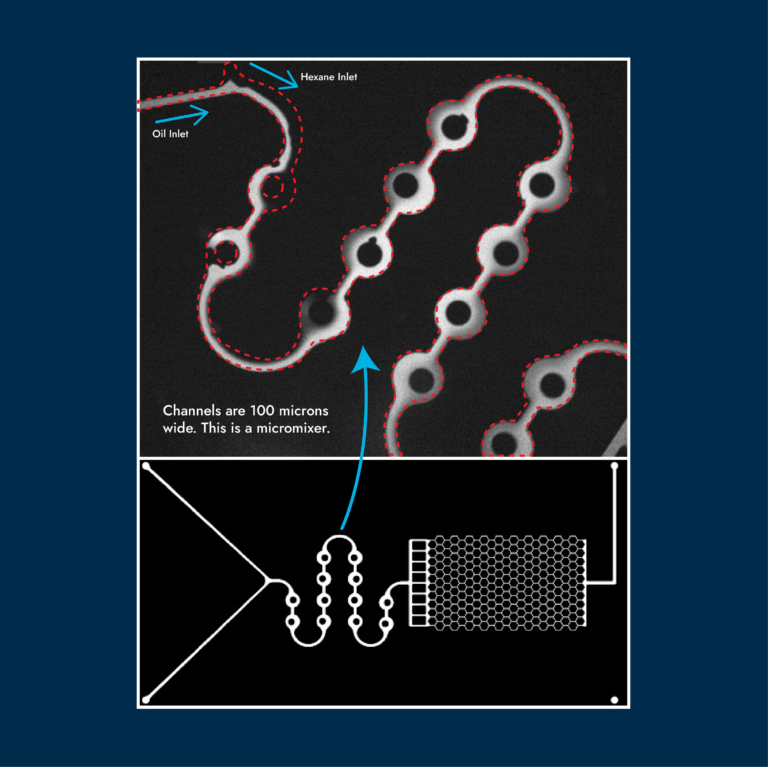
Fluid mixing in Interface’s miscibility testing
Interface often runs minimum miscibility pressure (MMP) tests for gas flooding clients, which involves diffusion and advection studies. MMP tests are one of the most sought-after Interface measurements, particularly by CO2-EOR clients.

Currently, slim-tube testing is the most widely used method for MMP measurement. However, it requires large samples and has a long turnaround time, making it extremely difficult to test the impact of a wide range of compositions.
In a slim-tube experiment, the Peclet number is ~2000, making the flow advection-dominated. With the Interface method, the Peclet number can be varied from ~400 to 4000 by adjusting the flow parameters. By doing so, Interface’s microfluidic method can match the dynamics of flow and mass transfer, similar to the slim-tube method.
Reynolds number, Schmidt number, and how they relate
There are two more important dimensionless numbers related to the Peclet number which are relevant to the work Interface does.
Reynolds number is perhaps one of the most well-known dimensionless numbers in fluid mechanics. Essentially, it predicts how fluids will flow depending on their density, viscosity, flow speed, and characteristic length. It can either describe laminar, sheet-like flow at lower values or turbulent flow at higher values.
Schmidt is another dimensionless number that characterizes fluid flow processes. It is the ratio of two different types of diffusion, namely, momentum and mass diffusion. Gas systems usually have the lowest Schmidt number, followed by gas-liquid and then high viscosity liquids.
When used in the context of species or mass transfer, the product of Reynolds and Schmidt numbers gives the Peclet number.
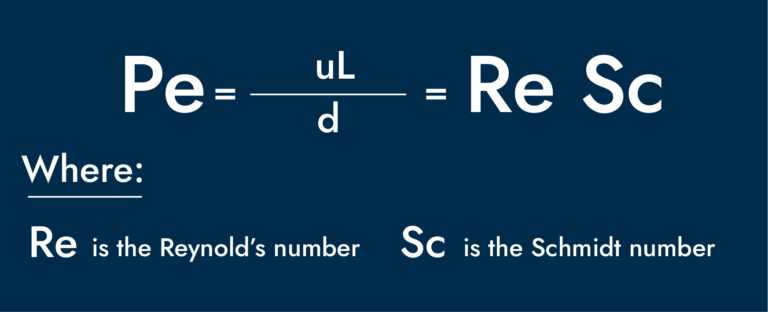
Overall, dimensionless numbers are important when comparing systems of different sizes. They are important parameters that inform Interface measurements. For more dimensionless number studies, check out our bond number and capillary pressure blog posts.